How to Find Critical Points
Since the critical points of a function come from the derivative of a function the derivative is the obvious way to finding the critical numbers. Free functions critical points calculator - find functions critical and stationary points step-by-step Upgrade to Pro Continue to site This website uses cookies to ensure you get the best experience.
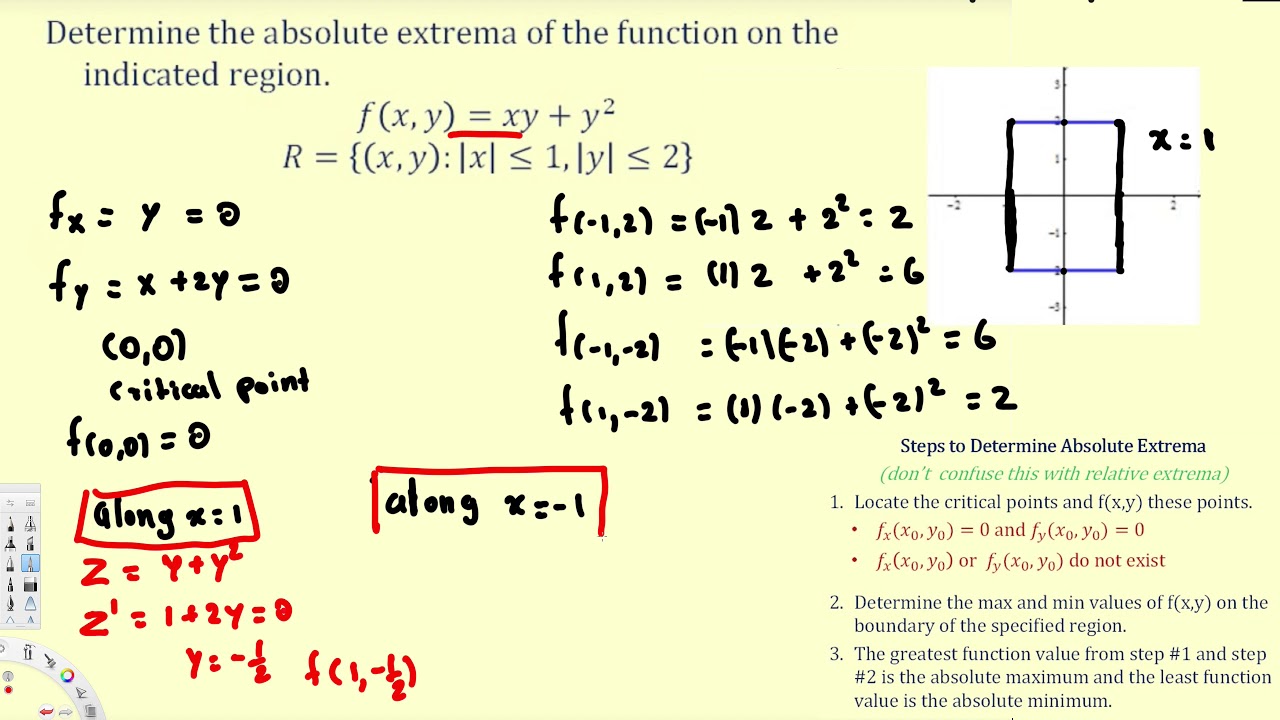
Absolute Extrema Of Functions Of Two Variables Vector Calculus Vector Calculus Calculus Extrema
Gt 3t22t1 g t t 2 3 2 t.

. Local minima at π2π2 π2π2 Local maxima. Example 2 Determine all the critical points for the function. Next find all values of the functions independent.
The critical points are then classified by employing the 2nd derivative test for. Note that f64 31. Consider a function eqf x eq.
In this video I show how to find the critical points by setting the first derivative eq. 2 Locate x values where f x 0 andor where f x is. Determining intervals on which a function is increasing or decreasing.
This is the currently selected item. Steps for finding the critical points of a given function f x. Equate the function derivate to zero and calculate for x.
Steps for finding the Critical points of a provided feature f x. This means that we will make Now solving for the second x. To find the critical points of a function y f x just find x-values where the derivative f x 0 and also the x-values where f x is not defined.
The method is to calculate the partial derivatives set them to zero and then solve to find the critical points. The only critical point 64. How to Find Critical Points of a Function.
Finding critical points can be essential in real-world applications such as finding out the area of a fenced-in yard or the lowest and highest points of a suspension bridge. This video focuses on how to find the critical points of a function. To find the critical points of a function first ensure that the function is differentiable and then take the derivative.
The graph of a function has either a horizontal tangent or a. 1 Take derivative of f x to get f x 2 Find x values where f x 0 andor where f x is undefined 3 Plug the values obtained. All local extrema and minima are the critical points.
The critical point is the tangent plane of points z f x y is horizontal or does not exist. Compute f xx 2f xy 4 and f yy 4 and so 24 42 0 at any point. 1 Take the by-product of f x to obtain f x.
The concept of critical point is very important in Calculus as it is used widely in solving optimization problems. Therefore at the critical point 6431 the surface has a saddle. So lets take a look at some functions that require a little more effort on our part.
That means that this function is constantly. The value or values computed will be the critical point or points. We need to first find the critical points f x 3 f x 3 Notice that this function has a constant derivative which is positive.

Critical Points Stationary Points And Inflection Point Calculus Urdu Hindi Inflection Point Calculus Pointe

Finding Critical Numbers Example 1 Math Videos Example Math

How Do You Find The Minimum And Maximum Values Of A Function Cool Math Tricks Math Tricks Fun Math

All Local Maximums And Minimums On A Function S Graph Called Local Extrema Occur At Critical Points Of The Function Wh Math Formulas Calculus Math Methods
0 Response to "How to Find Critical Points"
Post a Comment